| Welcome | People | Research | Presentations | Publications | Facilities | Links |
|---|
|
|
|
|
|
|
Efficient and Accurate Models for Electrokinetic Microfluidic Systems
![]() The objective of this project is to develop efficient and accurate physical models for electrokinetically based biofluidic components and use them to perform system-level simulations and synthesis to generate optimal designs that demonstrates 100 ~ 100,000x speedup over full numerical computations and trial-and-error experiments.
These models are efficient and accurate, and will ultimately lead to a CAD tool using the bottom-up iterative design methodology for the multi-functional and integrated lab-on-a-chip (LOC) systems.
A few highlights of this project are given below.
The objective of this project is to develop efficient and accurate physical models for electrokinetically based biofluidic components and use them to perform system-level simulations and synthesis to generate optimal designs that demonstrates 100 ~ 100,000x speedup over full numerical computations and trial-and-error experiments.
These models are efficient and accurate, and will ultimately lead to a CAD tool using the bottom-up iterative design methodology for the multi-functional and integrated lab-on-a-chip (LOC) systems.
A few highlights of this project are given below.
![]()
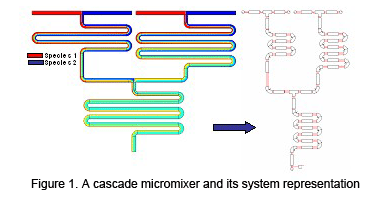
![]() Figure 1 shows a schematic of cascade micromixer that can be decomposed into reservoirs, channels and converging intersections. These elements are linked at their interfaces through two types of parameters.
These include the electric potential determined by Kirchhoff's and Ohm's laws, and sample concentration coefficients given by solutions of the convection-diffusion equation.
Analytical formulas are obtained for the individual elements, and are assembled to form a model for the complex mixer. The model agrees with results from full numerical simulation within 2%, while allowing a 250x speedup.
Figure 1 shows a schematic of cascade micromixer that can be decomposed into reservoirs, channels and converging intersections. These elements are linked at their interfaces through two types of parameters.
These include the electric potential determined by Kirchhoff's and Ohm's laws, and sample concentration coefficients given by solutions of the convection-diffusion equation.
Analytical formulas are obtained for the individual elements, and are assembled to form a model for the complex mixer. The model agrees with results from full numerical simulation within 2%, while allowing a 250x speedup.
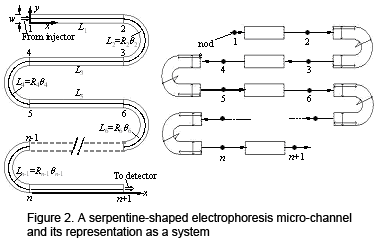
![]() Figure 2 shows a schematic of a serpentine-shaped electrophoretic separation microchannel. An analyte band migrating under electrophoresis will experience dispersion (or broadening of the band) due to
diffusion and non-uniformities in migration velocity, which can be caused by such factors as channel curvature and Joule heating. We have developed an efficient and accurate system-level model for
describing dispersion in such complex-shaped microchannels. Similar to the case of micromixers, the model consists of component-level physical models (e.g., for describing curvature and Joule heating-induced dispersion)
for individual channel elements of simple shapes (e.g., straight channels and semi-circular turns), which are assembled in such a way that neighboring elements are linked at their interface by a minimal set of physical parameters
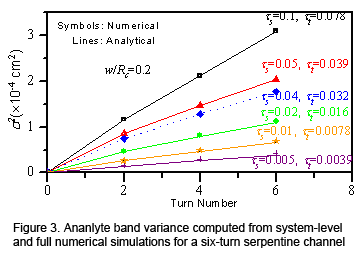
(e.g., the electric potential, separation time, analyte band variance, and coefficients representing the shape of a skewed analyte band) (Figure 2). We have validated the system-level model with both experiments and full numerical simulations,
with worst-case discrepancies ranging from < 1% to 9.5% depending on the dominance of convection in the dispersion process (Figure 3). A speedup of up to 15,000x has been observed for our model over full numerical simulations.
Figure 2 shows a schematic of a serpentine-shaped electrophoretic separation microchannel. An analyte band migrating under electrophoresis will experience dispersion (or broadening of the band) due to
diffusion and non-uniformities in migration velocity, which can be caused by such factors as channel curvature and Joule heating. We have developed an efficient and accurate system-level model for
describing dispersion in such complex-shaped microchannels. Similar to the case of micromixers, the model consists of component-level physical models (e.g., for describing curvature and Joule heating-induced dispersion)
for individual channel elements of simple shapes (e.g., straight channels and semi-circular turns), which are assembled in such a way that neighboring elements are linked at their interface by a minimal set of physical parameters
(e.g., the electric potential, separation time, analyte band variance, and coefficients representing the shape of a skewed analyte band) (Figure 2). We have validated the system-level model with both experiments and full numerical simulations,
with worst-case discrepancies ranging from < 1% to 9.5% depending on the dominance of convection in the dispersion process (Figure 3). A speedup of up to 15,000x has been observed for our model over full numerical simulations.
![]()
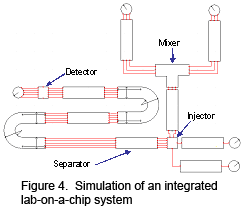
We are currently also developing a circuit-like framework to allow efficient and accurate simulations of an entire electrokinetic microfluidic system including a wide spectrum of subsystems such as mixers, reactors, separators,
along with injectors and detectors (Figure 4). Preliminary results have shown that the circuit-like simulation can be accomplished within one second of computational time, demonstrating the excellent promise of our simulation approach to complex LOC systems.
Dr. James Hoburg (Department of ECE, CMU)
Dr. Steinar Hauan (Department of ChemE, CMU)
Researchers
Yi Wang , Ph.D. Student (ME)